Formula For Calculating Volume In Chemistry

Using this calculator, you can calculate the molar volume of a gas for arbitrary temperature and pressure. Just note that for big values real gases divert from ideal gas law (that's why they are not "ideal") and this formula can't be used. Eventually, these individual laws were combined into a single equation—the ideal gas law—that relates gas quantities for gases and is quite accurate for low pressures and moderate temperatures. We will consider the key developments in individual relationships , then put them together in the ideal gas law.

The behavior of gases can be described by several laws based on experimental observations of their properties. The pressure of a given amount of gas is directly proportional to its absolute temperature, provided that the volume does not change (Amontons's law). The volume of a given gas sample is directly proportional to its absolute temperature at constant pressure (Charles's law). The volume of a given amount of gas is inversely proportional to its pressure when temperature is held constant (Boyle's law).

Under the same conditions of temperature and pressure, equal volumes of all gases contain the same number of molecules (Avogadro's law). A disadvantage of describing formulas as w/v (%) is that the description says nothing about the actual concentration of molecules in solution. What if we want equal amounts of two chemicals to be mixed together, so that for each molecule of substance #1 there is a single molecule of substance #2? The same amount in grams will likely not contain the same number of molecules of each substance.

Another disadvantage of the w/v method is that the same chemical can come in many forms, so that the same amount in grams of one form of the chemical contains a different amount of it than another form. For example, you may work with a chemical that can be in one of several forms of hydration. Calcium chloride can be purchased as a dry chemical in anhydrous form, so that what you weigh out is nearly all pure calcium chloride. On the other hand you may have a stock of dry chemical that is hydrated with seven water molecules per molecule of calcium chloride. The same mass of this chemical will contain fewer molecules of calcium chloride.

When we are interested in the actual concentration of molecules of a chemical in solution, it is better to have a universal measurement that works regardless of how the chemical is supplied. As long as the molecular weight is known, we can describe a solution in the form of moles per liter, or simply molar . Density, ratio of the mass of a substance to its volume, expressed, for example, in units of grams per cubic centimeter or pounds per cubic foot.

The density of a pure substance varies little from sample to sample and is often considered a characteristic property of the substance. Most substances undergo expansion when heated and therefore have lower densities at higher temperatures. Many substances, especially gases, can be compressed into a smaller volume by increasing the pressure acting on them. For these reasons, the temperature and pressure at which the density of a substance is measured are usually specified. The density of a gas is often converted mathematically to what it would be at a standard temperature and pressure . Water is unusual in that it expands, and thus decreases in density, as it is cooled below 3.98'C .

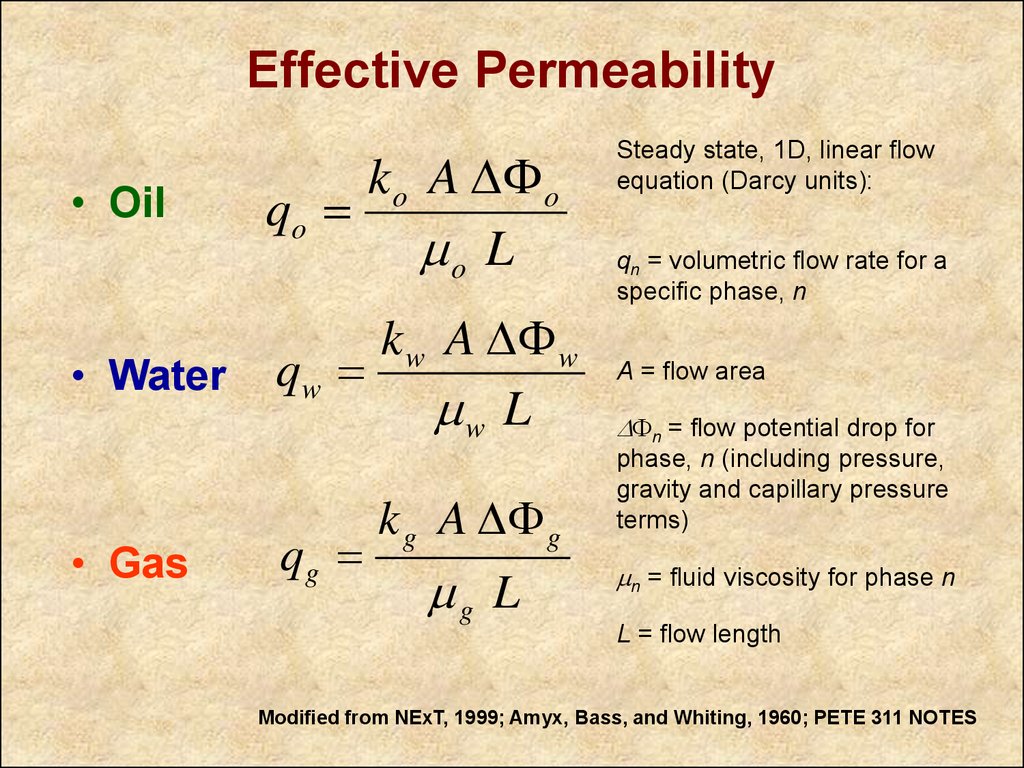
And is a proportionality constant that relates the values of pressure, volume, amount, and temperature of a gas sample. The variables in this equation do not have the subscripts i and f to indicate an initial condition and a final condition. The ideal gas law relates the four independent properties of a gas under any conditions.

Formula For Finding Volume In Chemistry As with w/v solutions, we weigh out a specific amount of chemical when making a molar solution. Unlike w/v solutions, the amount to weigh depends on the molecular weight (m.w.) of the substance in grams per mole (g/mol). In order to calculate the desired mass of solute you will need to know the formula weight. Formula weights are usually printed on the label and identified by the abbreviation f.w. Formula weight is the mass of material in grams that contains one mole of substance, and may include inert materials and/or the mass of water molecules in the case of hydrated compounds. For pure compounds the formula weight is the molecular weight of the substance and may be identified as such.

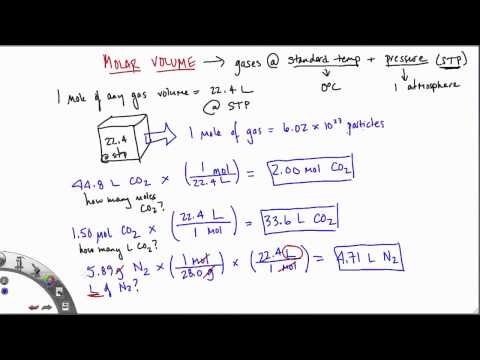
One mole of an ideal gas has a volume of 22.4 L at standard temperature and pressure. For more accurate measurements, glassware that has been certified by standards agencies may be purchased. The glass volumes are also calculated for the standard temperature of 20 °C, with small adjustments for borosilicate glass expansion or contraction with temperature changes.

Gases whose properties of P, V, and T are accurately described by the ideal gas law are said to exhibit ideal behavior or to approximate the traits of an ideal gas. An ideal gas is a hypothetical construct that may be used along with kinetic molecular theory to effectively explain the gas laws as will be described in a later module of this chapter. Although all the calculations presented in this module assume ideal behavior, this assumption is only reasonable for gases under conditions of relatively low pressure and high temperature.

In the final module of this chapter, a modified gas law will be introduced that accounts for the non-ideal behavior observed for many gases at relatively high pressures and low temperatures. To have value, measurement results must be metrologically traceable to an appropriate reference, which in the cases treated in this chapter are SI units of mass, volume, and amount of substance. A statement of measurement uncertainty always accompanies a traceable result. Methods must be validated and verified for use by a particular operator at a particular time.

Accreditation to an appropriate standard, such as ISO , is overseen by organisations usually with governmental or quasi-governmental status. Gaining accreditation for a particular method shows that a laboratory is using validated methods by competent personnel, but of course can never guarantee a reliable result . In this section we will review the components of measurement uncertainty of mass and volume measurements and then apply this to the preparation of a standard solution and a typical titration.

It is noted that the metrological traceability chain will involve multiple branches , often through amount fraction or mass fraction. For more information, see the chapter on quality assurance in the forthcoming 4th edition of the Orange Book , or in . Molar volume of a gas can be defined as volume of gas occupied by one mole of gas at 1 atm pressure and 273K temperature.

If you use ideal gas equation volume of one mole of gas will be 22.4L. Suppose that someone has already worked out the details, so all that you have to do is read a formula and make a solution. We can usually assume that a solution is to be aqueous unless stated otherwise. What about the concentration of the substance to be added?

Common ways of describing the concentrations of solutions are weight-in-weight, weight-in-volume, volume-in-volume, and molarity. Less commonly used descriptions include normality and molality. A quantity of solute is measured out, mixed with solvent, and the volume is brought to some final quantity after the solute is completely dissolved.

That is, solutions are typically prepared volumetrically. Because solutes add volume to a quantity of solvent, this method of preparation of solutions is necessary to ensure that an exact desired concentration is obtained. It is important to note that the molarity is defined as moles of solute per liter of solution, not moles of solute per liter of solvent. This is because when you add a substance, perhaps a salt, to some volume of water, the volume of the resulting solution will be different than the original volume in some unpredictable way. To get around this problem chemists commonly make up their solutions in volumetric flasks. These are flasks that have a long neck with an etched line indicating the volume.

The solute is added to the flask first and then water is added until the solution reaches the mark. The flasks have very good calibration so volumes are commonly known to at least four significant figures. Specific volume is defined as the number of cubic meters occupied by one kilogram of matter. It is the ratio of a material's volume to its mass, which is the same as the reciprocal of its density. In other words, specific volume is inversely proportional to density. Specific volume may be calculated or measured for any state of matter, but it is most often used in calculations involving gases.

Is the volume occupied by one mole of a chemical element or a chemical compound. It can be calculated by dividing the molar mass by mass density (ρ). Molar gas volume is one mole of any gas at a specific temperature and pressure has a fixed volume.

To apply this gas law, the amount of gas should remain constant. As with the other gas laws, the temperature must be expressed in kelvins, and the units on the similar quantities should be the same. Because of the dependence on three quantities at the same time, it is difficult to tell in advance what will happen to one property of a gas sample as two other properties change. The best way to know is to work it out mathematically. The interest stems from that accurate measurements of the unit cell volume, atomic weight and mass density of a pure crystalline solid provide a direct determination of the Avogadro constant. Perhaps you cannot find a formula weight on a label or perhaps you are planning a protocol and do not have the actual chemicals on hand.

You can calculate molecular weight from the chemical formula with the aid of a periodic table. You must keep in mind that when you purchase the chemical the formula weight may not be identical to the molecular weight. Suppose that you have already determined how much to weigh out based on the molecular weight, but the formula weight is greater due to hydration or the presence of inert material. Your remedy is simply to multiply your calculated mass by the ratio of formula weight to molecular weight . When we describe a concentration as a percentage without specifying the type of formula, we imply that the solution is to be made using the weight-in-volume (w/v) method. As with w/w, weight-in-volume is a simple type of formula for describing the preparation of a solution of solid material in a liquid solvent.

Solutions that require materials from natural sources are often prepared w/v because the molecular formula of the substance is unknown and/or because the substance cannot be described by a single formula. Perhaps the easiest way to describe a solution is in terms of weight-in-weight (w/w). The weight of the solute relative to the weight of the final solution is described as a percentage. For example, suppose you have a dye that is soluble in alcohol.

Rather than write the instructions, "take 3 grams dye and mix with 97 grams absolute alcohol," you can describe the solutions simply as 3% dye in absolute alcohol. The formula applies to any volume of solution that might be required. Three grams dye plus 97 grams alcohol will have final weight of 100 grams, so the dye winds up being 3% of the final weight. Note that the final weight is not necessarily equal to the final volume. Aqueous weight-in-weight solutions are the easiest to prepare. Since 1 milliliter of water weighs one gram, we can measure a volume instead of weighing the solvent.

A very common use of w/w formulas is with media for the culture of bacteria. Such media come in granular or powdered form, often contain agar, and often require heat in order to dissolve the components. Microbiological media, especially when they contain agar, are difficult to transfer from one vessel to another without leaving material behind.

They coat the surfaces of glassware, making quite a mess. Using a w/w formula the media and water can be mixed, heated, then sterilized, all in a single container. Very little material is wasted and there is less of a mess.

An aqueous solution consists of at least two components, the solvent and the solute . Usually one wants to keep track of the amount of the solute dissolved in the solution. One could do by keeping track of the concentration by determining the mass of each component, but it is usually easier to measure liquids by volume instead of mass. Molarity is defined as the number of moles of solute divided by the volume of the solution in liters.

The measurement of mass is a central point of the quantification of material substances. A balance measures mass by sensing the weight force that presses an object down on the balance pan. Weight is the force exerted on a body by the gravitational field of the earth, and is measured in the unit force newton, N. The weight force acting on 1 kg mass depends on geographic and cosmic factors. However, for mass measurements using mechanical balances, the weight of the unknown object is equilibrated at the same place and same time as the weight of an object of known mass (i.e. of a standard).

For high precision measurements, the buoyancy caused by the surrounding air must be taken into consideration. This correction can easily be calculated if the density of the known and unknown mass and that of the air is known. This relationship between temperature and pressure is observed for any sample of gas confined to a constant volume. An example of experimental pressure-temperature data is shown for a sample of air under these conditions in Figure 9.11. Volume is the quantification of the three-dimensional space a substance occupies.

By convention, the volume of a container is typically its capacity, and how much fluid it is able to hold, rather than the amount of space that the actual container displaces. Volumes of many shapes can be calculated by using well-defined formulas. In some cases, more complicated shapes can be broken down into simpler aggregate shapes, and the sum of their volumes is used to determine total volume.

The volumes of other even more complicated shapes can be calculated using integral calculus if a formula exists for the shape's boundary. Beyond this, shapes that cannot be described by known equations can be estimated using mathematical methods, such as the finite element method. Alternatively, if the density of a substance is known, and is uniform, the volume can be calculated using its weight. This calculator computes volumes for some of the most common simple shapes.

For regular three-dimensional objects, you can easily calculate the volume by taking measurements of its dimensions and applying the appropriate volume equation. If it's an irregular shape, you can try to do the very thing that caused Archimedes to shout the famous word Eureka! Probably you heard that story - Archimedes was asked to find out if the Hiero's crown is made from pure gold or just gold-plated - but without bending or destroying it.